In the last two posts (RTT and 60/40/20), we looked at how implied volatility (IV) and price of the option strikes in two broken wing butterfly (BWB) strategies changed with time. In this post, we'll look at another BWB strategy, the centered delta neutral (CDN) BWB. In this strategy, the short put options are at-the-money (ATM), the lower long is at least 100 points below the market, and the upper long is positioned to create a delta neutral structure. An SPX January 2018 expiration CDN is modeled below.
 |
| (click to enlarge) |
As in the last two articles, we'll use five option chains in our analysis. The options chains we'll use expire on:
- 03-Nov-2017 (7 DTE)
- 10-Nov-2017 (14 DTE)
- 17-Nov-2017 (20 DTE)
- 15-Dec-2017 (48 DTE)
- 19-Jan-2018 (83 DTE)
 |
| (click to enlarge) |
So what happens with the price of these put options as DTE decrease? They all lose value with time...not a surprise! The options at-the-money (ATM) lose the most...again, not a surprise. The upper longs in-the-money (ITM) lose the least. Similar to the IV chart above, the strikes of our CDN along with the current market price are marked with vertical lines.
 |
| (click to enlarge) |
For the lower long strike of our CDN, the 2470 strike, we have IVs at 7 DTE, 14 DTE, 20 DTE, 48 DTE, and 83 DTE. At 83 DTE the IV of the 2470 strike is 12.6%, and at 7 DTE the IV of the 2470 strike is 16.1%. The chart below shows how the price of the 2470 strike decays with variable IV (changing from 12.6% to 16.1%), with fixed IV of 12.6%, and with fixed IV of 16.1%. The variable IV (purple line) is closer to how this option price will actually decay.
 |
| (click to enlarge) |
The theoretical decay of the center short strike is shown in the chart below.
 |
| (click to enlarge) |
Finally, the theoretical decay of the upper long strike is shown in the next chart.
 |
| (click to enlarge) |
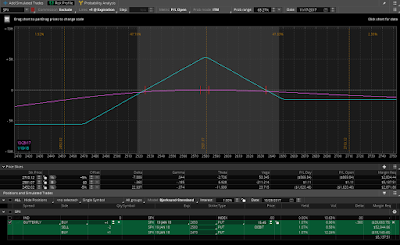
In ThinkOrSwim (TOS), using four 20 day steps, we can see how the price of the CDN changes with time. This is shown in the image below. We can see that if the market did not move, and if the IV stayed constant, we would expect the price to increase to expiration.
 |
| (click to enlarge) |
Using the theoretical Black-Scholes option prices from the analysis above, we can model the CDN price by DTE. Assuming the market and IV remain constant, the Black-Scholes model shows the CDN price change by DTE in the chart below.
 |
| (click to enlarge) |
Neither the TOS Bjerksund-Stensland model nor the Black-Scholes model reflect what will actually happen with this trade, even if both the SPX and IV remained constant. These models do provide a view of the general trend of price change with DTE, which can be useful when evaluating your actual trades.
This is all for now for BWB, but in the future we'll look more at how initial conditions impact the outcome of these trades.
Follow my blog by email, RSS feed or Twitter (@DTRTrading). All options are available on the top of the right hand navigation column under the headings "Subscribe To RSS Feed", "Follow By Email", and "Twitter".
No comments:
Post a Comment